
In OEIS: - A002878 Bisection of Lucas sequence. In OEIS: - A001906 F(2n) = bisection of Fibonacci sequence. The number of words of length n in alphabet ( xj w ) = 0 for i/=j).Sequences from the OEIS that pass the recursion test, but are not defined as recursions, are collected on a separate page: Non Recursions.

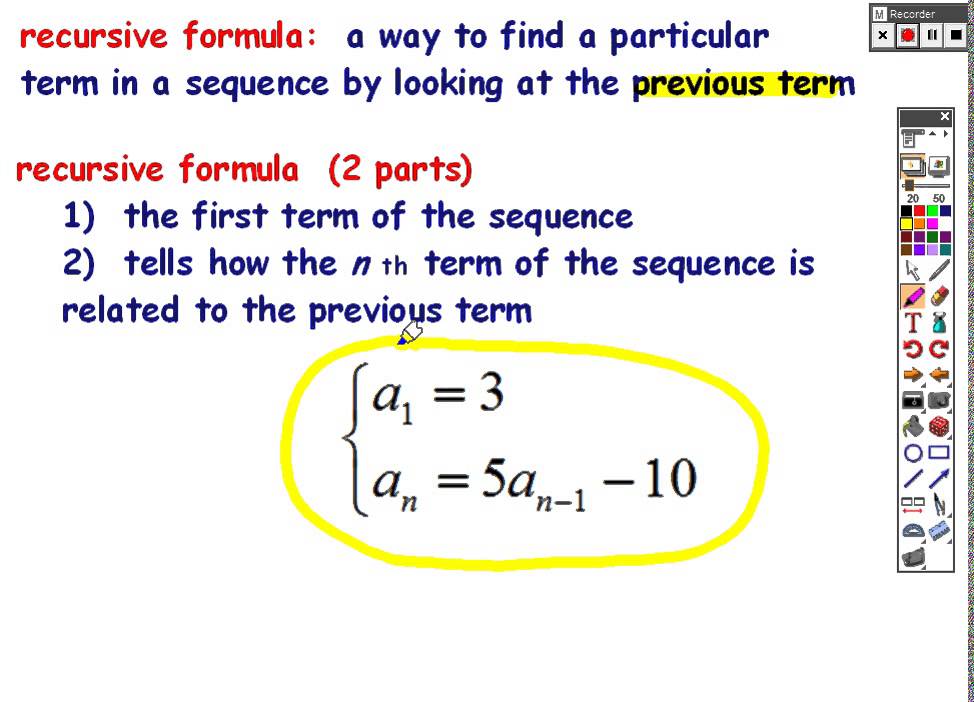
Sequences in OEIS may start with a different index. This page is synchronized with OEIS in January 2007. Maybe these having two levels of numbers to calculate the current number would imply that it would be some kind of quadratic function just as if I only had 1 level, it would be linear which is easier to calculate by hand. This gives us any number we want in the series. I do not know any good way to find out what the quadratic might be without doing a quadratic regression in the calculator, in the TI series, this is known as STAT, so plugging the original numbers in, I ended with the equation:į(x) = 17.5x^2 - 27.5x + 15. Then the second difference (60 - 25 = 35, 95-60 = 35, 130-95=35, 165-130 = 35) gives a second common difference, so we know that it is quadratic. = a ( 4 ) + 2 =a(4)+2 = a ( 4 ) + 2 equals, a, left parenthesis, 4, right parenthesis, plus, 2 = 9 =\goldD9 = 9 equals, start color #e07d10, 9, end color #e07d10Ī ( 5 ) a(5) a ( 5 ) a, left parenthesis, 5, right parenthesis = 7 + 2 =\blueD 7+2 = 7 + 2 equals, start color #11accd, 7, end color #11accd, plus, 2 = a ( 3 ) + 2 =a(3)+2 = a ( 3 ) + 2 equals, a, left parenthesis, 3, right parenthesis, plus, 2 = 7 =\blueD 7 = 7 equals, start color #11accd, 7, end color #11accdĪ ( 4 ) a(4) a ( 4 ) a, left parenthesis, 4, right parenthesis = 5 + 2 =\purpleC5+2 = 5 + 2 equals, start color #aa87ff, 5, end color #aa87ff, plus, 2 = a ( 2 ) + 2 =a(2)+2 = a ( 2 ) + 2 equals, a, left parenthesis, 2, right parenthesis, plus, 2 = 5 =\purpleC5 = 5 equals, start color #aa87ff, 5, end color #aa87ffĪ ( 3 ) a(3) a ( 3 ) a, left parenthesis, 3, right parenthesis = a ( 1 ) + 2 =a(1)+2 = a ( 1 ) + 2 equals, a, left parenthesis, 1, right parenthesis, plus, 2

= 3 =\greenE 3 = 3 equals, start color #0d923f, 3, end color #0d923fĪ ( 2 ) a(2) a ( 2 ) a, left parenthesis, 2, right parenthesis = a ( n − 1 ) + 2 =a(n\!-\!\!1)+2 = a ( n − 1 ) + 2 equals, a, left parenthesis, n, minus, 1, right parenthesis, plus, 2Ī ( 1 ) a(1) a ( 1 ) a, left parenthesis, 1, right parenthesis A ( n ) a(n) a ( n ) a, left parenthesis, n, right parenthesis


 0 kommentar(er)
0 kommentar(er)
